RBSE Solutions for Class 8 Maths Chapter 7 Construction of Quadrilaterals Ex 7.6 is part of RBSE Solutions for Class 8 Maths. Here we have given Rajasthan Board RBSE Class 8 Maths Chapter 7 Construction of Quadrilaterals Exercise 7.6.
| Board | RBSE |
| Textbook | SIERT, Rajasthan |
| Class | Class 8 |
| Subject | Maths |
| Chapter | Chapter 7 |
| Chapter Name | Construction of Quadrilaterals |
| Exercise | Exercise 7.6 |
| Number of Questions | 7 |
| Category | RBSE Solutions |
Rajasthan Board RBSE Class 8 Maths Chapter 7 Construction of Quadrilaterals Ex 7.6
Question 1.
Construct a square in which :
(i) Perimeter is 20 cm.
(ii) Sum(RBSESolutions.com)of two adjacent sides is 9 cm.
Solution
(i) Given that perimeter of a square = 20 cm.
∴ side of a square = \(\frac { Perimeter }{ 4 }\)
= \(\frac { 20 }{ 4 }\) = 5cm.
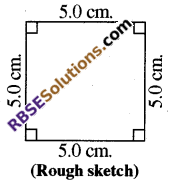
We draw a square whose side = 5 cm.
We know that each angle of a square = 90°
Steps of Construction
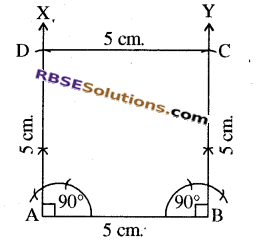
(1) First(RBSESolutions.com)of all draw a line segment AB = 5 cm.
(2) At the point A and B, construct ∠BAX = 90° and ∠ABY = 90° respectively with AB.
(3) From ray AX cuts AD = 5 cm. and from ray by cuts BC = 5 cm.
(4) Join CD.
Thus, we obtained required square ABCD.
(ii) Draw a rough sketch of square of given measurements.
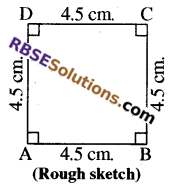
Given that
Sum of two adjacent sides = 9 cm.
So each(RBSESolutions.com)side of square = \(\frac { 9 }{ 2 }\) = 4.5 cm.
Steps of Construction
(1) First of all we draw a line segment AB = 4.5 cm.
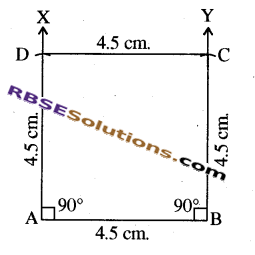
(2) At the point A and B we construct ∠BAX = 90° and ∠ABY = 90° with AB respectively.
(3) Similarly from point B, an angle of 90° in made and arc of 4.5 cm is cut. Here, mark this point as C.
(4) Join C and D.
Thus, we(RBSESolutions.com)obtained a square ABCD.
![]()
Question 2.
Construct a square PQRS in which diagonals meets at point O and PO = 2.8 cm.
Solution
First of all we draw a rough sketch of square ABCD of given measurements.
By properties of square we know that diagonals of a square are equal at bisect each other,
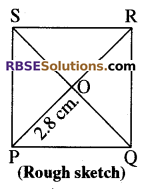
∵PO = 2.8 cm.
∴PR = 2 x PO
= 2 x 2.8
= 5.6 cm.
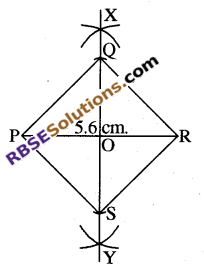
Steps of Construction
(i) We draw a line segment of diagonal PR = 5.6 cm.
(ii) Draw(RBSESolutions.com)perpendicular bisector XY of PR which intersect at point O.
(iii) Taking O as centre, draw an arc of radius 2.8 cm., both sides of PR which cuts perpendicular bisector XY at Q and S.
(iv) Join PQ, QR, RS and SP.
(v) Thus, we obtained required square PQRS.
Question 3.
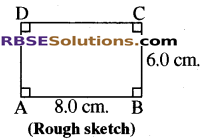
Construct a rectangle while adjacent sides are 8 cm. and 6 cm. respectively.
Solution
First of all we draw a rough sketch of the given measurements :
Steps of Construction
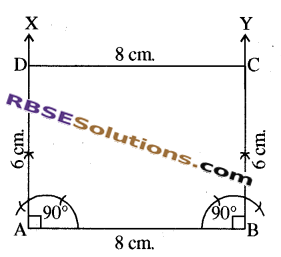
(i) First of all we draw a line segment AB = 8 cm.
(ii) At point A construct ∠BAX = 90° with AB.
(iii) From AX cut AD = 6 cm., an arc of 6.0 cm is cut on AX and marked a point D.
(iv) Similarly at(RBSESolutions.com)point B draw ∠ABY = 90° with AB and cut BC = 6 cm from ray BY.
(v) Join CD.
Thus, we obtained required rectangle ABCD.
![]()
Question 4.
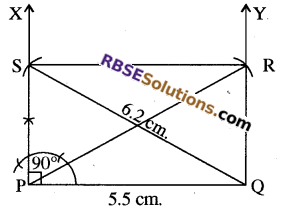
Construct a rectangle PQRS while PQ = 5.5 cm. and diagonal QS = 6.2 cm.
Solution
Draw a rough sketch of given measurements :
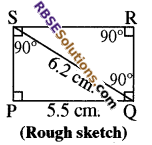
From rectangle properties each angle of a rectangle is 90° and opposite sides are equal and parallel to each other and diagonals are also equal to each other.
Steps of Construction
(i) First of all draw a line segment PQ = 5.5 cm.
(ii) At points P and Q construct ∠QPX = 90° and ∠PQY = 90° with PQ respectively.
(iii) At point P, draw an arc(RBSESolutions.com)of radius 6.2 cm. intersecting ray QY at point R. Join PR.
(iv) At point Q, draw an arc of radius 6.2 cm. intersecting ray PX at S. Join QS and SR. Thus, we obtained a rectangle PQRS.
![]()
Question 5.
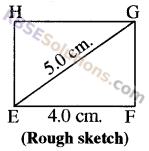
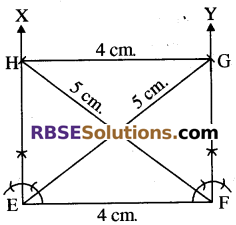
Construct a rectangle EFGH while EF = 4.0 cm. and diagonal EG = 5.0 cm.
Solution
First of all we draw a rough sketch of given measurements :
Steps of Construction
(i) First of all we draw a line segment EF = 4.0 cm.
(ii) At point E and F construct ∠FEX = 90° and ∠EFY = 90° with EF respectively with rules and compass only.
(iii) An arc of 5 cm. from(RBSESolutions.com)point E cut on FY and marked the point as G.
(iv) Similarly, an arc of 5 cm. is cut on EX and marked this point at H.
(v) Join EG, FG and GH.
Thus, we obtained required rectangle EFGH.
![]()
Question 6.
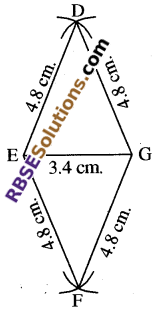
Construct a rhombus DEFG while FG = 4.8 cm and diagonal EG = 3.4 cm.
Solution
First of all we draw a rough sketch of given measurement :
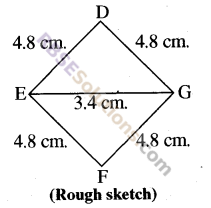
Steps of Construction
(i) First of all we draw a diagonal EG = 3.4 cm.
(ii) At point E and G, we draw two arc of radius 4.8 cm. and 4.8 cm. both side of EG intersecting each other at point D and F respectively.
(iii) Join ED, GD, GF and EF.
Thus, we obtained required rhombus DEFG.
![]()
Question 7.
Construct a rhombus ABCD while BC = 4.0 cm. and ∠B = 75°.
Solution
First of all we draw a rough sketch of given measurement :
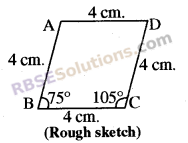
In the case of parallelogram :
∠B + ∠C = 180°
∠C = 180° – ∠B
= 180° – 75°
∠C = 105°
Steps of Construction
(i) We draw a line BC = 4 cm.
(ii) At point B and C respectively we construct ∠CBX = 75° and ∠BCY = 105° with BC with the help of ruler and compass only.
(iii) From(RBSESolutions.com)point B and C, two arc of each 4 cm. are cut on BX and CY. Mark these point A and D respectively.
(iv) Join AD.
Thus, we obtained required rhombus ABCD.

![]()
We hope the given RBSE Solutions for Class 8 Maths Chapter 7 Construction of Quadrilaterals Ex 7.6 will help you. If you have any query regarding Rajasthan Board RBSE Class 8 Maths Chapter 7 Construction of Quadrilaterals Exercise 7.6, drop a comment below and we will get back to you at the earliest.