RBSE Solutions for Class 9 Maths Chapter 13 Angles and their Measurement is part of RBSE Solutions for Class 9 Maths. Here we have given Rajasthan Board RBSE Class 9 Maths Solutions Chapter 13 Angles and their Measurement.
| Board | RBSE |
| Textbook | SIERT, Rajasthan |
| Class | Class 9 |
| Subject | Maths |
| Chapter | Chapter 13 |
| Chapter Name | Angles and their Measurement |
| Exercise | Ex 13 |
| Number of Questions Solved | 15 |
| Category | RBSE Solutions |
Rajasthan Board RBSE Class 9 Maths Solutions Chapter 13 Angles and Their Measurement
Multiple Choice Questions
Question 1.
The line describing (RBSESolutions.com) an angle of 750°, lies in:
(A) First quadrant
(B) Second quadrant
(C) Third quadrant
(D) Fourth quadrant
Question 2.
The number of radians in angle 30° is:
(A) \(\frac { \pi }{ 3 }\) radian
(B) \(\frac { \pi }{ 4 }\) radian
(C) \(\frac { \pi }{ 6 }\) radian
(D) \(\frac { 3\pi }{ 4 }\) radian
![]()
Question 3.
The value of \(\frac { 3\pi }{ 4 }\) in sexagesimal system is:
(A) 75°
(B) 135°
(C) 120°
(D) 220°
Question 4.
The time taken by the minute hand of (RBSESolutions.com) a watch in tracing an angle of \(\frac { \pi }{ 6 }\) radians is:
(A) 10 minutes
(B) 20 minutes
(C) 15 minutes
(D) 5 minutes
Question 5.
The value of the angle, in radian subtended at the centre of the circle of radius 100 metres by an arc of length 25π metres is:
(A) \(\frac { \pi }{ 4 }\)
(B) \(\frac { \pi }{ 3 }\)
(C) \(\frac { \pi }{ 6 }\)
(D) \(\frac { 3\pi }{ 4 }\)
Answers
1. A
2. C
3. B
4. D
5. A
Question 6.
In which quadrant does the (RBSESolutions.com) revolving ray lie when it makes the following angles.
(i) 240°
(ii) 425°
(iii) – 580°
(iv) 1280°
(v) – 980°
Solution.
(i) 240° = 2 x right angle + 60°, therefore the position of the revolving ray will be in third quadrant.
(ii) 425° = 4 x right angles + 65°, therefore the position of the revolving ray will be in first quadrant.
(iii) – 580° = – 6 x right angles – 40°, therefore the position of the revolving ray will be in second quadrant.
(iv) 1280° = 14 x right angles + 20°, therefore the position of the revolving ray will be in third quadrant.
(v) – 980° = – 10 x right angles – 80°, therefore the position of the revolving ray will be in second quadrant.
Question 7.
Convert the following angles (RBSESolutions.com) in radians:
(i) 45°
(ii) 120°
(iii) 135°
(iv) 540°
Solution.
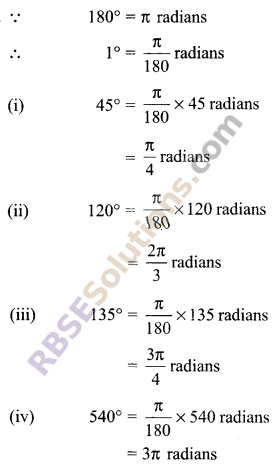
![]()
Question 8.
Express the following angles (RBSESolutions.com) in sexagesimal system.
(i) \(\frac { \pi }{ 2 }\)
(ii) \(\frac { 2\pi }{ 5 }\)
(iii) \(\frac { 5\pi }{ 6 }\)
(iv) \(\frac { \pi }{ 15 }\)
Solution.
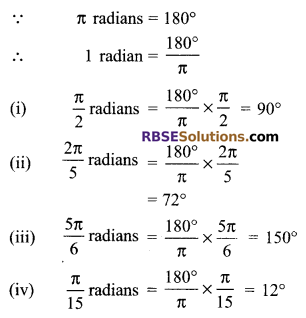
Question 9.
Find the angle in radians subtended at the centre of (RBSESolutions.com) a circle of radius 5 cm by an arc of the circle whose length is 12 cm.
Solution.
We know that:
θ (radian) = \(\frac { arc length }{ radius }\)
We have, radius = 5 cm
and arc length = 12 cm
θ = \(\frac { 12 }{ 5 }\)
Question 10.
How much time the minute hand of a watch will take to describe an angle of \(\frac { 3\pi }{ 2 }\) radians.
Solution.
Time taken by the minute hand of a watch in tracing 4 right angles or an angle equal to 2it radians = 1 hour.
Time taken by the minute hand of a clock in tracing an angle equal to 1 radian = \(\frac { 1 }{ 2\pi }\) hours
Time taken by the minute hand of a clock in tracing an angle equal to \(\frac { 3\pi }{ 2 }\) radians
= \(\frac { 1 }{ 2\pi } \times \frac { 3\pi }{ 2 }\) hours
= \(\frac { 3 }{ 4 }\) hours or 45 minutes 4
Question 11.
How much time the minute hand of a watch will take (RBSESolutions.com) to describe an angle of 120°?
Solution.
We know that:
The minute hand of a watch describes an angle of 4 right angles i.e. 360° in one hour.
the time taken by minute hand to trace an angle of 1° = \(\frac { 1 }{ 360 }\) hours
the time taken by minute hand to trace 120° angle
= \(\frac { 1 }{ 360 }\) x 120
= \(\frac { 1 }{ 3 }\) hours
= \(\frac { 1 }{ 3 }\) x 60 minutes
= 20 minutes.
Question 12.
Find the radius of the circle, if any arc length of 10 cm subtends (RBSESolutions.com) an angle of 60° at the centre of the circle.
Solution.
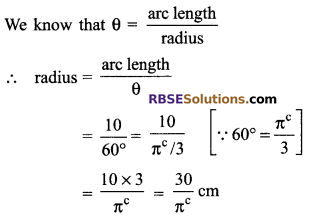
Question 13.
Find the time if the minute hand of a clock (RBSESolutions.com) has revolved through 30 right angles just after noon.
Solution.
We know that:
The time taken by the minute hand of a clock in tracing 4 right angles is 1 hour.
So, we convert 30 right angles in terms of the multiple of 4 right angles
i.e. 30 right angles
= 7 x (4 right angles) + 2 right angles
= 7 x 1 hr + \(\frac { 1 }{ 2 }\) hr = 7\(\frac { 1 }{ 2 }\) hours
= 7 hours 30 minutes
Hence, time = 7 : 30 p.m.
![]()
Question 14.
The angles of a triangle are in (RBSESolutions.com) the ratio of 2 : 3 : 4. Find all the three angles in radians.
Solution.
The A, B and C are angles of any triangle ABC
A : B : C = 2 : 3 : 4
⇒ ∠A = 2x, ∠B = 3x and ∠C = 4x
∠A + ∠B + ∠C = 180° (Angles sum property of a triangle)
⇒ 2x + 3x + 4x = 180°
⇒ 9x = 180°
⇒ x = 20°
Therefore angles (in degrees) are 40°, 60′ and 80°

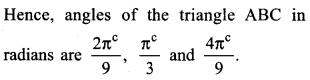
Question 15.
Convert \(\frac { 3\pi }{ 5 }\) radian into (RBSESolutions.com) sexagesimal system.
Solution.
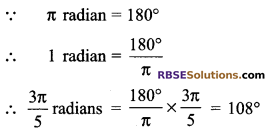
We hope the given RBSE Solutions for Class 9 Maths Chapter 13 Angles and their Measurement will help you. If you have any query regarding Rajasthan Board RBSE Class 9 Maths Solutions Chapter 13 Angles and their Measurement, drop a comment below and we will get back to you at the earliest.