RBSE Solutions for Class 9 Maths Chapter 3 Polynomial Ex 3.5 is part of RBSE Solutions for Class 9 Maths. Here we have given RBSE Rajasthan Board Solutions for Class 9 Maths Chapter 3 Polynomial Ex 3.5.
| Board | RBSE |
| Class | Class 9 |
| Subject | Maths |
| Chapter | Chapter 3 |
| Chapter Name | Polynomial |
| Exercise | Ex 3.5 |
| Number of Questions Solved | 12 |
| Category | RBSE Solutions |
RBSE Solutions for Class 9 Maths Chapter 3 Polynomial Ex 3.5
Question 1.
Use suitable identities to find (RBSESolutions.com) the following products:
(i) (x + 3)(x + 7)
(ii) (x – 5)(x + 8)
(iii) (2x + 7)(3x – 5)
(iv) (5 – 3x)(3 + 2x)
(v) \(\left( { x }^{ 2 }+\frac { 3 }{ 5 } \right) \left( { x }^{ 2 }-\frac { 3 }{ 5 } \right) \)
(vi) (x + 2)(x – 5)
Solution.
(i) ∵ (x + a)(x + b) = x2 + (a + b)x + ab
∴(x + 3)(x + 7) = x2 + (3 + 7)x + 3 x 7
= x2 + 10x + 21
(ii) ∵ (x + a)(x + b) = x2 + (a + b)x + ab
∴(x + (- 5))(x + 8)
= x2 + (- 5 + 8)x + (- 5) x (8)
= x3 + 3x – 40
(iii) We have, (2x + 7)(3x + 5)

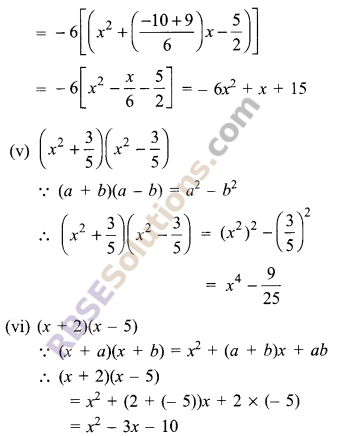
![]()
Question 2.
Evaluate the following (RBSESolutions.com) products without multiplying directly.
(i) 104 x 109
(ii) 94 x 97
(iii) 103 x 97
Solution.
(i) 104 x 109 = (104 + 4)(100 + 9)
Using identity
(x + a)(x + b) = x² + (a + b)x + ab, we get
104 x 104
= (100)² + (4 + 9) x 100 + 4 x 9
= 10000 + 1300 + 36 = 11336
(ii) 94 x 97 = (100 – 6)(100 – 3)
= (100)² + [(- 6) + (- 3)] x 100 + (- 6) x (- 3)
= 10000 – 900 + 18 = 9118
(iii) 103 x 97 = (100 + 3)(100 – 3)
Using identity
(a + b)(a – b) = a² – b², we get
103 x 97 = (100)² – (3)²
= 10000 – 9 = 9991
Question 3.
Factorise the following (RBSESolutions.com) by using suitable identity
(i) x² + 6xy + 9y²
(ii) x² – 4x + 4
(iii) \(\frac { { x }^{ 2 } }{ 100 } \) – y²
Solution.
(i) x² + 6xy + 9y²
= (x)² + 2.x.3y + (3y)²
= (x + 3y)² = (x + 3y)(x + 3y)
(ii) x² – 4x + 4
= (x)² – 2.x.2 + (2)²
= (x – 2)²
= (x – 2)(x – 2)
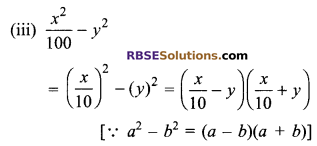
Question 4.
Expand each of the following, using (RBSESolutions.com) suitable identity.
(i) (2a – 3b – c)²
(ii) (2 + x – 2y)²
(iii) (a + 2b + 4c)²
(iv) (m + 2n – 5p)²
(v) (3a – 7b – c)²
(vi) \({ \left( \frac { x }{ y } +\frac { y }{ z } +\frac { z }{ x } \right) }^{ 2 }\)
Solution.
(i) We have, (2a – 3b – c)²
Using identity (a + b + c)²
= a² + b² + c² + 2ab + 2bc + 2ca, we get
∴(2a – 3b – c)²
= (2a)² + (- 3b)² + (-c)² + 2(2a)(- 3b) + 2(- 3b)(- c) + 2(- c)2a
= 4a² + 9b² + c² – 12ab + 6bc – 4ac
(ii) We have, (2 + x – 2y)²
= (2)² + (x)² + (- 2y)² + 2(2)(x) + 2(x)(- 2y) + 2(2)(- 2y)
= 4 + x² + 4y² + 4x – 4xy – 8y
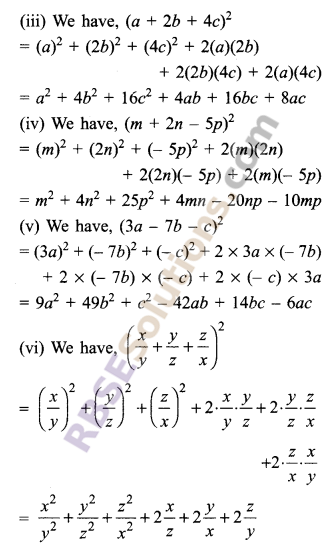
![]()
Question 5.
Factorise:
(i) 9x² + 4y² + 16z² – 12xy – 16yz + 24xz
(ii) x² + 2y² + 8z² + 2√2xy – 8yz – 4√2xz
Solution.
(i) We have,
9x² + 4y² + 16z² – 12xy – 16yz + 24xz
= (3x)² + (- 2y)² + (4z)² + 2(3x)(- 2y) + 2(- 2y)(4z) + 2(3x)(4z)
[∵ a² + b² + c² + 2ab + 2bc + 2ca = (a + b + c)²]
= (3x – 2y + 4z)²
= (3x – 2y + 4z)(3x – 2y + 4z)
(ii) We have,
x² + 2y² + 8z² + 2√2xy – 8yz – 4√2xz = (x)² + (√2y)² + (- 2√2z)² + 2(x)(√2y) + 2(√2y)(- √2z) + 2(x)(- 2√2z)
[∵ a² + b² + c² + 2ab + 2bc + 2ca = (a + b + c)²]
= (x + √2y – 2√2z)²
= (x + √2y – 2√2z)(x + √2y – 2√2z)
Question 6.
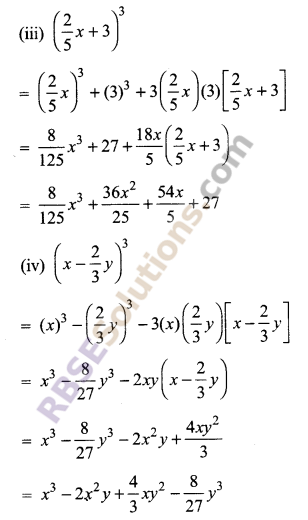
Expand the following:
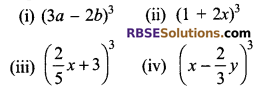
Solution.
(i) (3a – 2b)3
= (3a)3 – (2b)3 – 3(3a)(2b) [3a – 2b]
= 27a3 – 8b3 – 18ab (3a – 2b)
= 27a3 – 8b3 – 54a2b + 36ab2
= 27a3 – 54a2b + 36ab2 – 8b3
(ii) (1 + 2x)3
= (1)3 + (2x)3 + 3(1)(2x)[1 + 2x]
= 1 + 8x3 + 6x (1 + 2x)
= 1 + 8x3 + 6x + 12x2
= 1 + 6x + 12x2 + 8x3
Question 7.
Find the value of the following (RBSESolutions.com) by using suitable identity.
(i) (98)3
(ii) (103)3
(iii) (999)3
Solution.
(i) (98)3
= (100 – 2)3
= (100)3 – (2)3 – 3(100)(2) [100 – 2]
= 1000000 – 8 – 60000 + 1200
= 941192
(ii) (103)3
= (100 + 3)3
= (100)3 + (3)3 + 3(100)(3) [100 + 3]
= 1000000 + 27 + 90000 + 2700
= 1092727
(iii) (999)3
= (1000 – 1)3
= (1000)3 – (1)3 – 3(1000)(1) [1000 – 1]
= 100000000 – 1 – 3000000 + 3000
= 997002999
![]()
Question 8.
Factorise the following:
(i) x3 + 8y3 + 6x2y + 12xy2
(ii) 27a3 – 8b3 – 54a2b + 36ab2
(iii) 27 – 125x3 – 135x + 225x2
(iv) 125x3 + 64y3 – 300x2y + 240xy2
Solution.
(i) x3 + 8y3 + 6x2y + 12xy2
= (x)3 + (2y)3 + 3(x)(2y) [x + 2y]
= (x + 2y)3
[∵ (a + b)3 = a3 + b3 + 3 ab(a + b)]
(ii) 27a3 – 8b3 – 54a2b + 36ab2
= (3a)3 – (2b)3 – 3(3a)(2b) [3a – 2b]
= (3a – 2b)3
[∵ (a – b)3 = a3 – b3 – 3ab(a – b)]
(iii) 27 – 125x3 – 135x + 225x2
= (3)3 – (5x)3 – 3(3)(5x) [3 – 5x]
= (3 – 5x)3
[∵ (a – b)3 = a3 – b3 – 3ab(a – b)]
(iv) 125x3 – 64y3 – 300x2y + 240xy2
= (5x)3 – (4y)3 – 3(5x)(4y) [5x – 4y]
= (5x – 4y)3
[∵ (a – b) = a3 – b3 – 3ab(a – b)]
Question 9.
Factorise the following:
(i) 64a3 + 21b3
(ii) 125x3 – 8y3
Solution.
(i) 64a3 + 27b3
= (4a)3 + (3b)3
= (4a + 3b)[(4a)2 – 4a.3b + (3b)2]
[∵ a3 + b3 = (a + b)(a2 – ab + b2)]
= (4a + 3b)[ 16a2 – 12a6 + 9b2]
(ii) 125x2 – 8y3
= (5x)3 – (2y)3
= (5x – 2y)[(5x)2 + 5x.2y + (2y)2]
[ a3 – b3 = (a – b)(a2 + ab + b2)]
= (5x – 2y)[25x2 + 10xy + 4y2]
Question 10.
Verify that (i) x3 + y3 + z3 – 3xyz
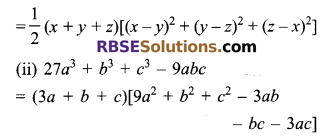
Solution.
(i) RHS

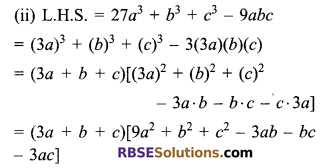
Question 11.
If x + y + z = 0, show that x3 + y3 + z3 = 3xyz.
Solution.
We are given that x + y + z = 0 and we know that
x3 + y3 + z3 – 3xyz
= (x + y + z)(x2 + y2 + z2 – xy – yz – zx)
Since x + y + z = 0, then
x3 + y3 + z3 – 3xyz
= 0 × (x2 + y2 + z2 – xy – yz – zx)
⇒ x3 + y3 + z3 – 3xyz = 0
⇒ x3 + y3 + z3 = 3xyz
Question 12.
Without actually calculating the cubes, find (RBSESolutions.com) the value of each of the following:
(i) (30)3 + (20)3 + (- 50)3
(ii) (- 15)3 + (28)3 + (- 13)3
Solution.
(i) (30)3 + (20)3 + (- 50)3
We know that a3 + b3 + c3 = 3abc, iff a + b + c = 0
Here 30 + 20 – 50 = 0 i.e. a + b + c = 0
⇒ (30)3 + (20)3 + (- 50)3
= 3 x (30) x (20) x (- 50)
= – 90000
(ii) (- 15)3 + (28)3 + (- 13)3
We know (RBSESolutions.com) that a3 + b3 + c3 = 3abc, if a + b + c = 0
Here a + b + c = – 15 + 28 + (- 13) = 0
(- 15)3 + (28)3 + (- 13)3
= 3 x (- 15) x 28 x (- 13)
= 16380
![]()
We hope the given RBSE Solutions for Class 9 Maths Chapter 3 Polynomial Ex 3.5 will help you. If you have any query regarding RBSE Rajasthan Board Solutions for Class 9 Maths Chapter 3 Polynomial Ex 3.5, drop a comment below and we will get back to you at the earliest.