RBSE Solutions for Class 9 Maths Chapter 5 Plane Geometry and Line and Angle Ex 5.2 is part of RBSE Solutions for Class 9 Maths. Here we have given Rajasthan Board RBSE Class 9 Maths Solutions Chapter 5 Plane Geometry and Line and Angle Ex 5.2.
| Board | RBSE |
| Class | Class 9 |
| Subject | Maths |
| Chapter | Chapter 5 |
| Chapter Name | Plane Geometry and Line and Angle |
| Exercise | Ex 5.2 |
| Number of Questions Solved | 11 |
| Category | RBSE Solutions |
Rajasthan Board RBSE Class 9 Maths Solutions Chapter 5 Plane Geometry and Line and Angle Ex 5.2
Question 1.
In the figure, lines AB, CD and EF are (RBSESolutions.com) parallel.
Find the angles ∠x, ∠y, ∠z and ∠p.
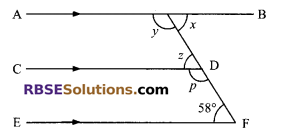
Solution.
∵ AB || CD || EF (given)
∴ ∠y + 58° = 180°
(interior angle sum)
⇒ ∠y = 180° – 58° = 122°
∴ ∠x = 180° – 122°
= 58° (linear pair)
Now AB || CD
∠x = ∠z = 58° (alt. angles) and ∠y = ∠p= 122°
(corresponding angles)
Hence, x = 58°, y = 122°, z = 58°, and
p = 122°.
![]()
Question 2.
In the given figure, AB || EF. Find ∠x and ∠y

Solution.

∵ AB || EF (given)
Also draw a line through P parallel to AB or EF
∴ 125°+ ∠APQ = 180°
(sum of the interior angles on the (RBSESolutions.com) same side of a transversal is 180°)
⇒ ∠APQ = 180°- 125°
⇒ ∠APQ = 55°
Also PQ || EF (by const.)
∠QPE + 141°= 180°
(reason as above )
⇒ ∠QPE = 39°
∴ ∠x = 55° + 39° = 94°
and ∠y = 360° – 94° = 266°
Question 3.
In figure, the line l || m then find the (RBSESolutions.com) angles which are equal to ∠1.
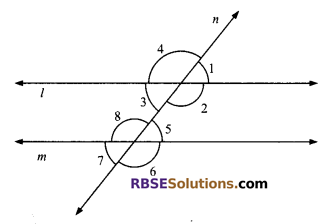
Solution.
∴ l || m
⇒ ∠1 = ∠3 (Vertically opposite angles)
Also ∠1 = ∠5 (Corresponding angles)
∠5 = ∠7 (Vertically opposite angles)
Hence, ∠3, ∠5 and ∠7 are equivalent to ∠1.
Question 4.
In the given figure, ∠1 = 60° and ∠6 = 120°, show (RBSESolutions.com) that m and n are parallel.
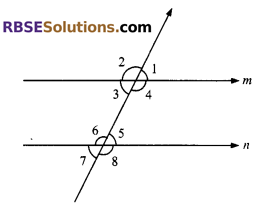
Solution.
∠1 = 60° (given)
∠3 = ∠1 = 60°
(vertically opposite angle)
and ∠6 = 120° (given)
∠3 + ∠6 = 60° + 120°
= 180°
i. e. sum of the interior angles on the (RBSESolutions.com) same side of transversal is 180°.
⇒ m || n.
Question 5.
If a transversal intersects two parallel lines l and m then prove that the bisectors AP and BQ of any two alternate angles are parallel i.e. AP || BQ.
Solution.
Given: l || m and a transversal intersect these two parallel lines at A and B respectively.
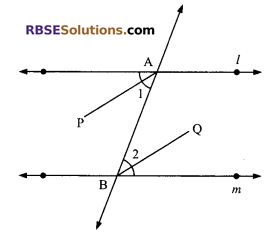
AP and BQ are the bisectors (RBSESolutions.com) of two alternate angles
To prove: AP || BQ
Proof: ∵ l || m (given)
⇒ ∠1 = ∠2
(alternate interior angles)
⇒ \(\frac { 1 }{ 2 }\)∠1 = \(\frac { 1 }{ 2 }\)∠2
⇒ ∠PAB = ∠QBA
Hence, the two lines AP and BQ are intersected by a transversal AB forming a pair of alternate angles equal.
∴AP || BQ Hence proved
![]()
Question 6.
In figure, BA || ED and BC || EF. Show (RBSESolutions.com) that ∠ABC + ∠DEF = 180°

Solution.
∵ BA || ED
∠1 = ∠2 …(i)
(Corresponding angles)
Also BC || EF
⇒ ∠2 + ∠3 = 180° ,..(ii)
(Sum of the interior angles on the (RBSESolutions.com) same side of a transversal is 180°)
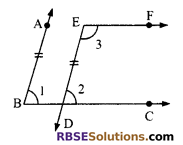
From (i) and (ii), we get
∠1 + ∠3 = 180°
i.e. ∠ABC + ∠DEF = 180°
Question 7.
In the figure, DE || QR and AP and BP are (RBSESolutions.com) the bisectors of ∠EAB and ∠RBA. Find the value of ∠APB.

Solution.
DE || QR (given)
∴∠EAB + ∠RBA = 180°
(Sum of the interior angles on (RBSESolutions.com) the same side of a transversal is 180°)
⇒ \(\frac { 1 }{ 2 }\)∠EAB + \(\frac { 1 }{ 2 }\)∠RBA = 90° …(i)
AP and BP are bisectors of ∠EAB and ∠RBA respectively
\(\frac { 1 }{ 2 }\)∠EAB = ∠PAB
\(\frac { 1 }{ 2 }\)∠RBA = ∠PAB
∵In ∆APB
∠PAB + ∠PBA + ∠APB = 180°

⇒ 90° + ∠APB = 180°
⇒ ∠APB = 90°
Question 8.
Two lines are perpendicular to two (RBSESolutions.com) parallel lines respectively. Show that these two lines are also parallel.
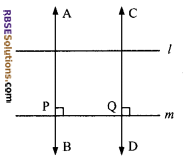
Solution.
∵ AB and CD are (RBSESolutions.com) perpendicular to l and m
∴∠APQ = 90° and ∠CQM = 90°
∠APQ = ∠CQM = 90°
(Corresponding- angles)
∴ AB || CD
Question 9.
In figure, If AB || CD, CD || EF and y : z = 3 : 7, find x.
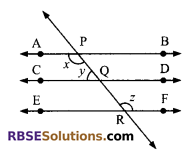
Solution.
We are given (RBSESolutions.com) that AB || CD and CD || EF
∴ ∠CQR = ∠QRF = z …(i)
(Alternate angles)
and ∠APQ = ∠CQR = x …(ii)
(Corresponding angles)
From (i) and (ii), we get
x = z …(iii)
Also ∠CQP + ∠CQR= 180°
(Linear pair)
⇒ y + z = 180°
But y : z = 3 : 7
⇒ y = 3k and z = 7k
⇒ 3k + 7k = 180°
10k = 180°
k = 18°
∴ y = 3k = 3 × 18° = 54°
and z = 7k = 7 × 18° = 126°
Hence. x = z = 126° [using (iii)]
![]()
Question 10.
In the figure, PQ and RS are two mirrors (RBSESolutions.com) placed parallel to each other. An incident ray AB strikes the mirror PQ at B, the reflected ray moves along the path BC and strikes the mirror RS at C and again reflects back along CD. Prove that AB || CD.
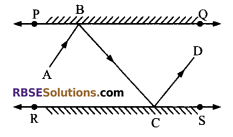
Solution.
At point B, draw BM ⊥ RS and (RBSESolutions.com) at point C, draw CN ⊥ PQ
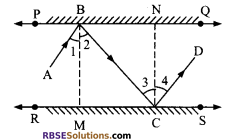
∠1 = ∠2 …(i)
and ∠3 = ∠4 ,..(ii)
(angle of incidence is equal to angle of reflection)
Also ∠2 = ∠3 …(iii)
(Alternate angles)
From (i), (ii) and (iii), we get
⇒ ∠1 = ∠4
⇒ 2∠1 = 2∠4
⇒ ∠1 + ∠1 = ∠4 + ∠4
⇒ ∠1 + ∠2 = ∠3 + ∠4
[using (i) and (ii)]
⇒ ∠ABC = ∠BCD
(Alternate angles)
⇒ AB || CD
Question 11.
In figure, if PQ || RS, ∠MXQ = 135° and ∠MYR = 40° then find ∠XMY.
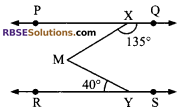
Solution.
Construction: Draw (RBSESolutions.com) a line || to PQ or RS through M
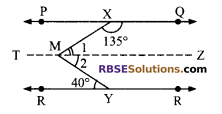
TZ || PQ
⇒ 135° + ∠1 = 180°
⇒ ∠1 = 180° – 135° = 45° …(i)
(Sum of interior angles on the (RBSESolutions.com) same side of a transversal is 180°)
Also RS || TZ …(ii)
⇒ ∠2 = 40° (alternate interior angle)
Adding (i) and (ii), we get
∠1 + ∠2 = 45° + 40°
= 85°
![]()
We hope the given RBSE Solutions for Class 9 Maths Chapter 5 Plane Geometry and Line and Angle Ex 5.2 will help you. If you have any query regarding RBSE Rajasthan Board Solutions for Class 9 Maths Chapter 5 Plane Geometry and Line and Angle Ex 5.2, drop a comment below and we will get back to you at the earliest.