RBSE Solutions for Class 9 Maths Chapter 7 Congruence and Inequalities of Triangles Additional Questions is part of RBSE Solutions for Class 9 Maths. Here we have given Rajasthan Board RBSE Class 9 Maths Solutions Chapter 7 Congruence and Inequalities of Triangles Additional Questions.
| Board | RBSE |
| Class | Class 9 |
| Subject | Maths |
| Chapter | Chapter 7 |
| Chapter Name | Congruence and Inequalities of Triangles |
| Exercise | Additional Questions |
| Number of Questions Solved | 32 |
| Category | RBSE Solutions |
Rajasthan Board RBSE Class 9 Maths Solutions Chapter 7 Congruence and Inequalities of Triangles Additional Questions
Multiple Choice Questions
Question 1.
In figure, if AB = AC and ∠B = 70°, the (RBSESolutions.com) value of ∠A will be:

(A) 70°
(B) 40°
(C) 55°
(D) 90°
Solution:
(B) 40°
Question 2.
In figure, if AB = AC, then the value of ∠C is equal to:
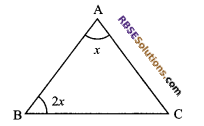
(A) 60°
(B) 36°
(C) 72°
(D) 108°
Solution:
(C) 72°
![]()
Question 3.
If the perpendicular drawn from the (RBSESolutions.com) mid-point of one side of a triangle to its other two sides are equal, then triangle is:
(A) Equilateral
(B) Isosceles
(C) Equiangular
(D) Scalene
Solution:
(B) Isosceles
Question 4.
In figure, AB = AC and AD ⊥ BC, then AD bisects the:
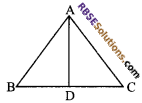
(A) ∠A
(B) Side BC
(C) ∠A and side BC
(D) None of these
Solution:
(C) ∠A and side BC
Question 5.
∆ABC, shown (RBSESolutions.com) in figure, in which AD = BD, and AC = DC and ∠C = 44°, then ∠A is:
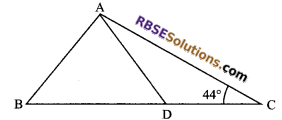
(A) 68°
(B) 112°
(C) 34°
(D) 102°
Solution:
(D) 102°
Question 6.
In an isosceles triangle, AB = AC and side BA is (RBSESolutions.com) produced up to D such that AB = AD, then ∠BCD is:
(A) 70°
(B) 90°
(C) 60°
(D) 45°
Solution:
(B) 90°
Question 7.
In figure, AB = AC and ∠ABD = ∠ACD then ∆BDC is:
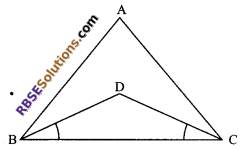
(A) Equilateral
(B) Isosceles
(C) Equiangular
(D) Scalene
Solution:
(B) Isosceles
Question 8.
In figure, AB = AC and AD is the (RBSESolutions.com) bisector of ∠BAC meet BC at D. If ∠BAC = 60° then ∠ADC is equal to:
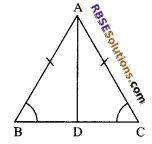
(A) 30°
(B) 60°
(C) 90°.
(D) 120°
Solution:
(C) 90°
Question 9.
In two triangles ABC and DEF, if AC = DF, BC = EF and ∠ABC = ∠DEF = 90° then two triangles are said to be congruent by:
(A) RHS property
(B) SAS property
(C) ASA property
(D) SSS property
Solution:
(A) RHS property
Question 10.
In the adjoining figure, if PQ = PR and QS = RT then ∆PST is equal to:
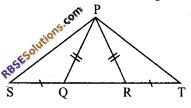
(A) Isosceles triangle
(B) Equilateral triangle
(C) Scalene triangle
(D) Isosceles (RBSESolutions.com) right angled triangle
Solution:
(A) Isosceles triangle
![]()
Very Short Answer Type Questions
Question 1.
In figure, AB = AC, CD = CA and ∠ADC = 20°, find ∠ABC.
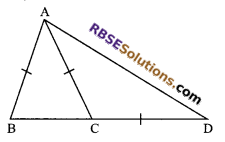
Solution.
CD = CA
⇒ ∠CAD = ∠ADC = 20°
⇒ ∠ACD = 180° – (20° + 20°) = 140°
∠ACB = 180° – 140° = 40°
But AB also equal to AC
⇒ ∠ABC = ∠ACB = 40°
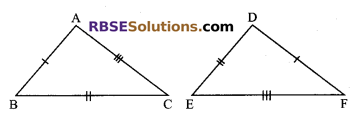
Question 2.
In Δ’s ABC and DEF, if AB = DF, BC = DE, AC = EF and ∠D = 55°. Find ∠B.
Solution.
According to above (RBSESolutions.com) information ΔABC = ΔDEF
(by c.p.c.t) ∠D = ∠B = 55°
Question 3.
In figure, ∠B = ∠D = 90° and BC = CD. Is AB = DE? Why?
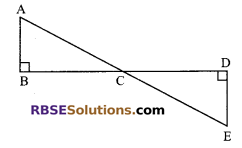
Solution.
In right angled triangles ABC and CDE
∠B = ∠D = 90° (given)
∠ACB = ∠DCE (vertically opposite angles)
and BC = CD (given)
ΔABC = ΔCDE (by ASA congruency property)
AB = DE (by c.p.c.t)
⇒ Yes, AB and DE are equal.
Question 4.
In a ΔABC, if ∠A = ∠B = 45°, which is the (RBSESolutions.com) longest side?
Solution.
∠A = ∠B = 45° (given)
∠C = 90°
Hence AB will be the longest side.
Question 5.
In figure, AB = 7 cm, BC = 8 cm and AC = 7.6 cm then write the
(i) greatest angle of the triangle
(ii) smallest angle (RBSESolutions.com) of the triangle
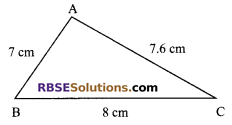
Solution.
(i) ∠CAB
(ii) ∠ACB
Question 6.
In ΔPQR, ∠Q = 35°, ∠R = 61° and the (RBSESolutions.com) bisector of ∠QPR meet QR at x. Then arrange the sides PX, QX and RX in descending order of their length.
Solution.
QX > PX > XR
Short Answer Type Questions
Question 1.
In the given figure, AB = AC and BD = EC then prove that ΔADE is an isosceles triangle.
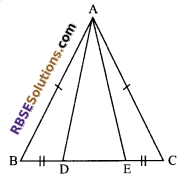
Solution.
In ΔABD and ΔAEC
AB = AC (given) …(i)
∠ABC = ∠ACB …(ii) (angle opposite to equal sides are equal)
Also BD = EC (given) …(iii)
From (i), (ii) and (iii), we have
ΔABD = ΔAEC (by SAS congruency property)
⇒ AD = AE (by c.p.c.t)
Hence, ΔADE is (RBSESolutions.com) an isosceles triangle.
Hence proved.
![]()
Question 2.
In figure, BA ⊥ AC, DE ⊥ EF, such that BA = DE and BF = CD, prove that AC = EF.
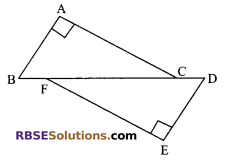
Solution.
To prove: AC = EF, we have to prove
ΔABC = ΔDEF
Here BA = DE (given) …(i)
BA ⊥ AC and DE ⊥ FE
∠BAC = ∠DEF = 90° (each) …(ii)
Also BF = CD (given) …(iii)
Adding FC in equation (iii), we get
BF + FC = CD + FC
⇒ BC = FD …(iv)
From (i), (ii) and (iv), we get
ΔABC = ΔDEF (by RHS congruency property)
⇒ AC = EF (by c.p.c.t)
Hence proved.
Question 3.
In figure, CB = AD and AB = CD. Can we say ∠ABC and ∠ADC are equal? (RBSESolutions.com) Why?

Solution.
In ΔABC and ΔADC
AB = DC
AD = CB
and AC = AC
ΔABC = ΔADC (by SSS congruency property)
So, ∠ABC = ∠ADC (by c.p.c.t)
Hence proved.
Question 4.
In figure, CN ⊥ AB, DM ⊥ AB and CN = DM. Are OC and OD equal? Why?
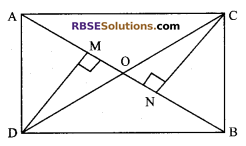
Solution.
In ΔOMD and ΔONC
∠OMD = ∠ONC (each 90°)
∠MOD = ∠NOC (vertically opposite angles)
and CN = DM (given)
ΔOMD = ΔONC (by AAS property)
⇒ OC = OD (by c.p.c.t)
Hence proved.
Question 5.
In figure, ∠A = 35°, ∠ABC = 100° and BD ⊥ AC, prove that ΔBDC is an isosceles triangle.
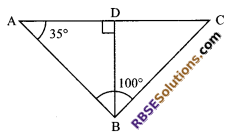
Solution.
In ΔABC,
∠A + ∠B + ∠C = 180° (the sum of the (RBSESolutions.com) interior angles of a Δ is equal to 180°)
35° + 100° + ∠C= 180°
⇒ ∠C = 180° – 135°
⇒ ∠C = 45°
But in ΔABD, BD ⊥ AC
i.e., ∠ADB = 90°
and ∠A = 35° (given)
∠ABD = 180° – 125° = 55°
∠DBC = 100° – 55° = 45°
Now, ∠DCB = ∠DBC = 45° (Proved above)
Hence BDC is an isosceles triangle.
Question 6.
In the given figure, O is the (RBSESolutions.com) middle point of both AB and CD. Prove that AC = BD and AC || BD.
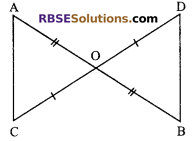
Solution.
In Δ’s AOC and BOD
OA = OB
OC = OD
∠AOC = ∠BOD (vertically opposite angles)
ΔAOC = ΔBOD (by SAS congruence property)
Hence AC = BD (by c.p.c.t)
Also ∠OAC = ∠OBD (alt. angle)
AC || BD
Hence proved.
![]()
Question 7.
In figure, X and Y are the points (RBSESolutions.com) on equal sides of AB and AC such that AX = AY. Show that XC = BY.
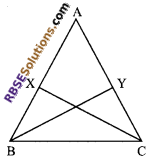
Solution.
In ΔABC
AB = AC (given)
and AX = AY (given)
⇒ AB – AX = AC – AY
⇒ BX = CY ….(i)
Now in ΔBXC and ΔBYC
BX = CY [from (i)]
BC = BC (common)
∠B = ∠C (angle opp. to equal sides are equal)
ΔBXC = ΔBYC (by SAS property)
⇒ XC = BY (by c.p.c.t)
Hence proved.
Question 8.
In the following figures, two sides (RBSESolutions.com) of ΔABC, AB and BC and median AD are respectively equal to PQ, QR and median PM of ΔPQR.
Prove that: ΔABC = ΔPQR.
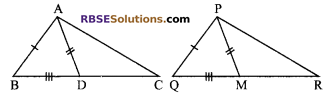
Solution.
According to question
AB = PQ (given)
Median AD = Median PM
and BC = QR
\(\frac { 1 }{ 2 }\) BC = \(\frac { 1 }{ 2 }\) QR
BD = QM
ΔABD = ΔPQM (by SSS congruency property)
∠B = ∠Q (by c.p.c.t)
Now in ΔABC and ΔPQR
AB = PQ (given)
∠B = ∠Q (proved above)
and BC = QR (given)
ΔABC = ΔPQR (by SAS congruency property)
Hence proved.
Question 9.
In figure, side QR of ΔPQR is (RBSESolutions.com) produced on both sides such that ∠PQS = ∠PRT. Prove that PQ = PR.
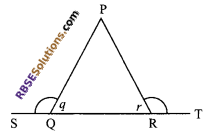
Solution.
In ΔPQR
∠PQS + ∠PQR = 180° (linear pair angles)
⇒ ∠PQS + ∠q = 180°
⇒ ∠q = 180° – ∠PQS …(i)
Also ∠r = 180° – ∠PRT …(ii)
But ∠PQS = ∠PRT (given) …(iii)
From (iii), eqn (i) becomes
∠q = 180° – ∠PRT …(iv)
Now from (ii) and (iv), we have
∠q = ∠r
i.e., ∠PQR = ∠PRQ
⇒ PR = PQ (converse of isosceles A theorem)
Hence proved.
Question 10.
Prove that the medians bisecting (RBSESolutions.com) the equal sides of an isosceles triangle are equal.
Solution.
Given: In ΔABC, D and E are mid-points of AB and AC respectively.
To prove: BE = CD
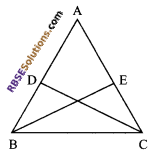
Proof: Since triangle ABC is (RBSESolutions.com) an isosceles triangle, then
AB = AC …(i)
and ∠ABC = ∠ACB …(ii)
D and E are mid-points of AB and AC respectively,
then DB = DA and EC = AE …(iii)
Now, in ΔBCD and ΔBCE
BC = BC (common)
∠DBC = ∠ECB by (ii)
BD = CE by (iii)
ΔBCD = ΔBCE (by SAS congruency rule)
⇒ BE = CD
Hence proved.
![]()
Long Answer Type Questions
Question 1.
“If two sides of a triangle are unequal (RBSESolutions.com) then the longer side has greater angle opposite to it.” Prove it.
Solution.
Given: A ΔABC in which AC > AB (say)
To prove: ∠ABC > ∠ACB
Construction: Mark a point D on AC such that AB = AD.
Join BD.
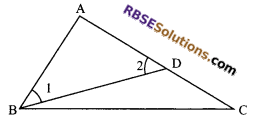
Proof: In ΔABD
AB = AD (by construction)
⇒ ∠1 = ∠2 …(i) (angles opposite to equal sides are equal)
Now in ΔBCD
∠2 > ∠DCB (ext. angle is greater than (RBSESolutions.com) one of the opposite interior angles)
⇒ ∠2 > ∠ACB …(ii) [∵ ∠ACB = ∠DCB]
From (i) and (ii), we get
∠1 > ∠ACB …(iii)
But ∠1 is a part of ∠ABC
∠ABC > ∠1 …..(iv)
Now from (iii) and (iv), we get
∠ABC > ∠ACB
Hence proved.
Question 2.
In figure, PQR is a triangle and S is (RBSESolutions.com) any point in its interior, show that SQ + SR < PQ + PR.

Solution.
Given: S is any point in the interior of ΔPQR
To prove: SQ + SR < PQ + PR
Construction: Produce QS to meet PR in T.
Proof: In ΔPQT PQ + PT > QT (sum of two sides of Δ is greater than third side)
or, PQ + PT > QS + ST …(i)
Also in ΔRST
ST + TR > RS …(ii)
Adding (i) and (ii), we get
PQ + PT + ST + TR > SQ + ST + SR
⇒ PQ + (PT + TR) > SQ + SR
⇒ PQ + PR > SQ + SR
Hence proved.
Question 3.
In figure, T is a point on side QR of ΔPQR and S is (RBSESolutions.com) a point such that RT = ST. Prove that PQ + PR > QS.
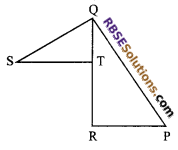
Solution.
In ΔPQR, we have
PQ + PR > QR (the sum of two sides of a triangle is greater than third side).
⇒ PQ + PR > QT + TR [∵ QT + TR = QR]
⇒ PQ + PR > QT + ST …(i) [∵ TR = ST]
In ΔQST, we have
QT + ST > QS …(ii)
From (i) and (ii), we get
PQ + PR > QS.
Hence proved.
Question 4.
In figure, side AB and AC are produced (RBSESolutions.com) to point D and E respectively. Bisectors BO and CO of ∠DBC and ∠ECB respectively meet at O. If AB > AC. Prove that OC > OB.
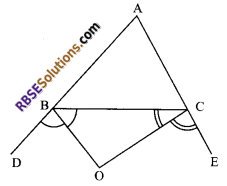
Solution.
Given: ABC is a triangle in which AB and AC is produced up to D and E respectively and bisectors of ∠DBC and ∠ECB meet at O. Also AB > AC.
To prove: OC > OB
Proof: In ΔABC
AB > AC
⇒ ∠ACB > ∠ABC …(i)
Also BO and CO are the bisectors of ∠DBC and ∠BCE respectively.
∠OBD = ∠OBC
and ∠OCB = ∠OCE
∠ACB = 180° – ∠BCE
⇒ ∠ACB = 180° – 2∠OCB …(ii)
Similarly ∠ABC = 180° – 2 ∠OBC…(iii)
From (i), (ii) and (iii), we have
180° – 2∠OCB > 180° – 2∠OBC
⇒ – 2∠OCB > – 2∠OBC
⇒ ∠OBC > ∠OCB
⇒ OC > OB
Hence proved.
![]()
Question 5.
If figure, ABCD is a quadrilateral. Prove that AB + BC + CD + DA > AC + BD.
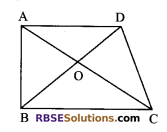
Solution.
In ΔABC
AB + BC > AC …(i) (the sum of (RBSESolutions.com) any two sides of a Δ is greater than third side)
Also in ΔADC
AD + DC > AC …(ii) (reason as above)
Similarly in ΔABD and ΔBCD
AB + AD > BD …(iii)
BC + CD >BD …(iv)
Adding (i), (ii), (iii) and (iv), we get
2AB + 2BC + 2AD + 2CD > 2AC + 2BD
⇒ AB + BC + CD + DA > AC + BD
Hence proved.
Question 6.
In the given figure, PQRS is a quadrilateral. PQ is its (RBSESolutions.com) longest side and RS is its shortest side. Prove that: ∠R > ∠P and ∠S > ∠Q.
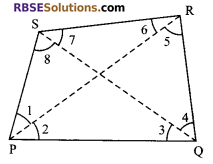
Solution.
Given: PQRS is a quadrilateral. PQ is its (RBSESolutions.com) longest side and RS is its shortest side.
To prove: ∠R > ∠P and ∠S > ∠Q
Construction: Join PR and QS.
Proof: In ΔPQR
PQ is longest side (given)
PQ > QR
⇒ ∠5 > ∠2 …(i) (∠ opposite to longer side is greater)
In ΔPSR
RS is the shortest side (given)
PS > RS
⇒ ∠6 > ∠1 …(ii)
Adding (i) and (ii), we get
∠5 + ∠6 > ∠1 + ∠2
⇒ ∠R > ∠P
Now in ΔPQS, PQ is the (RBSESolutions.com) longest side
PQ > PS
⇒ ∠8 > ∠3 …(iii)
In ΔSRQ, RS is the shortest side
RQ > RS …(iv)
⇒ ∠7 > ∠4
Adding (iii) and (iv), we get
∠7 + ∠8 > ∠3 + ∠4
∠S > ∠Q
Hence proved.
We hope the given RBSE Solutions for Class 9 Maths Chapter 7 Congruence and Inequalities of Triangles Additional Questions will help you. If you have any query regarding RBSE Rajasthan Board Solutions for Class 9 Maths Chapter 7 Congruence and Inequalities of Triangles Additional Questions, drop a comment below and we will get back to you at the earliest.