RBSE Solutions for Class 9 Maths Chapter 7 Congruence and Inequalities of Triangles Ex 7.1

RBSE Solutions for Class 9 Maths Chapter 7 Congruence and Inequalities of Triangles Ex 7.1 is part of RBSE Solutions for Class 9 Maths. Here we have given Rajasthan Board RBSE Class 9 Maths Solutions Chapter 7 Congruence and Inequalities of Triangles Exercise 7.1

Board	RBSE
Class	Class 9
Subject	Maths
Chapter	Chapter 7
Chapter Name	Congruence and Inequalities of Triangles
Exercise	Ex 7.1
Number of Questions Solved	10
Category	RBSE Solutions

Rajasthan Board RBSE Class 9 Maths Solutions Chapter 7 Congruence and Inequalities of Triangles Ex 7.1

Question 1.
In ∆ABC and ∆PQR, ∠A = ∠Q and ∠B = ∠R. Then which side of ∆PQR is equal (RBSESolutions.com) to side AB of ∆ABC. So that both triangles become congruent. Give reason for your answer.

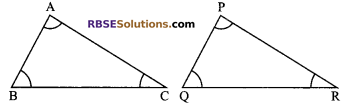
Solution.
In ∆ABC and ∆PQR
∠A = ∠Q, ∠B = ∠R then side AB should equal to QR
i.e., AB = QR
∴ ∆AOC ≅ ∆PQR (by ASA)

Question 2.
In triangles, ABC and PQR, ∠A = ∠Q and ∠B = ∠R then which side of ∆PQR is equal (RBSESolutions.com) to side BC of ∆ABC, so that both triangles congruent? Give reasons for your answer.

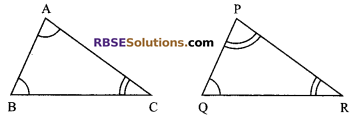
Solution.
In ∆ABC and ∆PQR
∠A = ∠Q
∠B = ∠R
=> ∠C = ∠P
(when two angles of ∆ABC and ∆PQR are equal then third angle of ∆ABC automatically equal to third angle of other ∆PQR)
PR = BC
=> ∆ABC ≅ ∆PQR (By ASA)

Question 3.
If two sides and one angle of a triangle are equal to two sides and (RBSESolutions.com) the angle of other then both the triangles must congruent to each other. Is this statement true?
Solution.
No, for any two triangles to be congruent it is must that any two sides and included angle of one is correspondingly equal to the other.

Question 4.
If two angles and one side of a triangle are equal to two angles and one side of other triangle then both A’s must congruent to each other. Is this statement true?
Solution.
No, side must be included between the angles.

Question 5.
If ∆ABC = ∆RPQ is given. It (RBSESolutions.com) is true to say BC = QR? and why?
Solution.
No, ∵ ∆ABC = ∆RPQ then BC should equal to PQ.

Question 6.
If ∆PQR = ∆EDF then is it true to say PR = EF? Give reasons for your answer.
Solution.
∵ ∆PQR ≅ ∆EDF
⇒ PR = EF
(these are corresponding sides)

Question 7.
In figure, the diagonal AC of a quadrilateral ABCD bisects (RBSESolutions.com) the angle A and C. Prove that AB = AD and CB = CD.

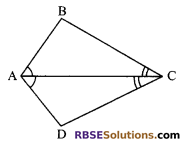
Solution.
In ∆ABC and ∆ADC
∠BAC = ∠DAC (given)
and ∠BCA = ∠ACD (given)
and side AC = side AC (common)
∴ ∆ABC ≅ ∆ADC
(by ASA congruency property)
⇒ AB = AD and CB = CD
(by c.p.c.t)
Hence proved.

Question 8.
In figure, ADBC is a quadrilateral (RBSESolutions.com) in which ∠ABC = ∠ABD and BC = BD then show that ∆ABC ≅ ∆ABD.

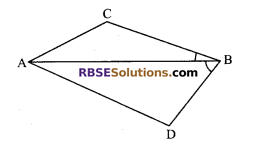
Solution.
In ∆ABC and ∆ABD
∠ABC = ∠ABD (given)
BC = BD (given)
AB = AB (common side)
⇒ ∆ABC ≅ ∆ABD
(By SAS congruency property)
Hence proved.

Question 9.
In figure, AB || CD and AD || BC then (RBSESolutions.com) show that ∆ADB ≅ ∆BCD.

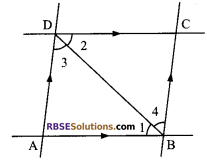
Solution.
In ∆ADB and ∆BCD
∵ AB || CD
⇒ ∠1 = ∠2 (alternate angles)
Also AD || BC
∠3 = ∠4 (alternate angle)
and BD = BD
∆ADB ≅ ∆BCD (common)
(by ASA congruency property)
Hence proved.

Question 10.
In figure, if AB || CD and E is the mid-point of AC, then (RBSESolutions.com) show that E is the mid-point of BD.

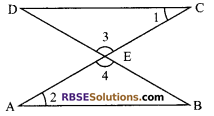
Solution.
In ∆AEB and ∆DEC
AB || CD
⇒ ∠1 = ∠2 (alternate angles)
∠3 = ∠4
(vertically opposite angles)
and AE = EC
(∵ E is the mid-point of AC)
⇒ ∆AEB ≅ ∆DEC
(by ASA congruency property)
Hence DE = EB (by c.p.c.t)
⇒ E is the mid-point of BD.

We hope the given RBSE Solutions for Class 9 Maths Chapter 7 Congruence and Inequalities of Triangles Ex 7.1 will help you. If you have any query regarding Rajasthan Board RBSE Class 9 Maths Solutions Chapter 7 Congruence and Inequalities of Triangles Exercise 7.1, drop a comment below and we will get back to you at the earliest.